A Rational System for Creating Order and Perfect Proportion in Architecture and Art Quizlet
Past Stacey Lewis
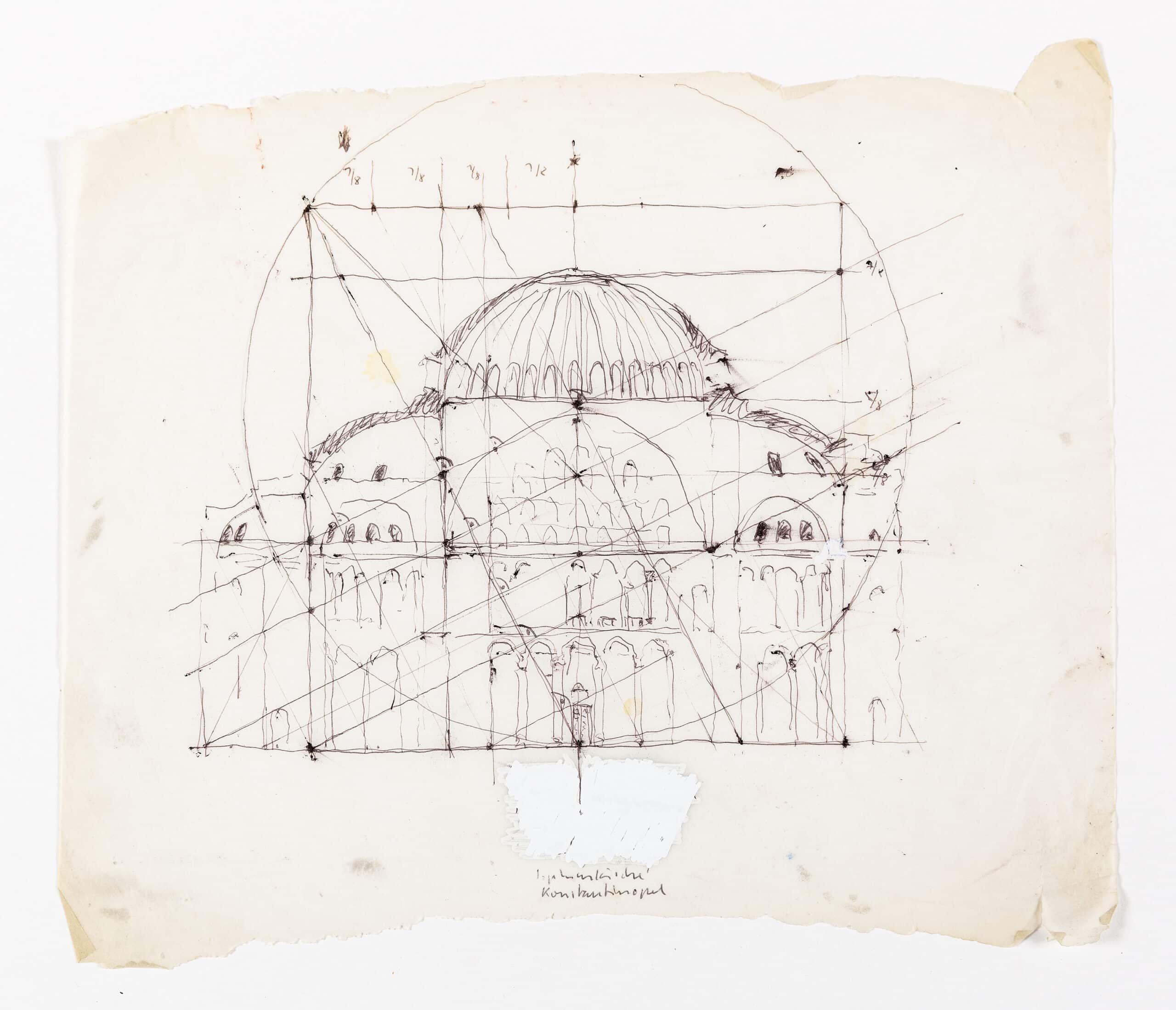
Peter Märkli's hand-fatigued section of the ancient monument Hagia Sophia (532–7) is role of a working procedure developed aslope his design work. The output is a collection of investigative drawings that document sacred archetypal buildings, and articulate his resolved thesis that 'architecture has a language'.
The weight of the drawing is in illustrating a system of proportion inherited from our earliest ancestors. For centuries, proportions were the foundation of the architectural discipline, used on an equal level for sacred, profane and domestic buildings. However, an increasing deviation from the practise of proportions has resulted in contemporary discourse to pause from the past, towards subjective theories of aesthetics. Märkli is aware of this shift, where, every bit he sees information technology, the universal language of compages is dissolving forth with homo ingenuity, in a society devoted to the service of a purely technical earth.
Märkli explains the linguistic communication of architecture through two overlapping principles: proportion and grammer. Proportion is a organization of measurement used to create harmony and rhythm between a building and its constituent parts. It is defined by the equality of ratios between ii pairs of quantities. That is to say, proportion determines space in 3-dimensions through two extremes, and a centre term, usually the mean. Grammar is the appropriate apply and refinement of architectural elements, notably the wall, platform, postal service, and roof. Proportion is therefore inherent to the building, and does not result from personal fancy, but from objective rules. Grammer is the refinement of a edifice to formalise the structure of a site, its genius loci, and expresses new meanings – meanings that are geographic and cosmic, such as history and memory, the climate, changing of seasons, the rise and setting of the sunday, and the difference betwixt dark and 24-hour interval. Furthermore, Märkli practices and teaches compages as a discipline. He believes an architect must train to see the language of compages before beginning to design; just every bit a musician trains to hear the sounds of music before beginning to etch [1].
The primeval expressions of a strict mathematical order are recorded in Arab republic of egypt and Babylon (2500–3000 BCE) in the form of pyramids, ziggurats and tomb chambers. Knowledge of Egyptian and Babylonian thinking is derived from sources drawn on papyrus and inscribed on clay tablets. These treatises draw mathematical inquires and reveal a procedure of design that was made visible in architectural form. A surviving co-operative of knowledge that transcends linguistic and temporal boundaries to universal questions of truth and reason.
Wisdom from Egypt and Babylon disseminated through maritime trade relations and influenced early on art and architecture that emerged in Ancient Greece. The evolution of the Greek polis (city-state) in the eighth century BCE gave ascension to independent political entities. The polis, particularly in Athens, became a setting for experiments in democracy, philosophy and science. A new class of free citizens began to rationally interpret the nature of the universe using mathematical logic. Past 550 BCE, through the teachings of the Greek philosopher Pythagoras, geometry had become a theoretical science.
Pythagoras and his followers believed the entire universe was ordered and governed by numbers, and their observations led to the discovery that music and mathematics share the same key basis. Information technology was this direct relation, between audible harmony in music, and visible order in mathematics, that constituted the chosen ratios and proportions found to be harmonious. In the first instance, this was based on first-manus feel of the sounds associated with numeric variations of pitch. This process crafted a twelve-tone musical scale consisting of five ratios; 12:6 (two:1), 9:6 (3:2), 8:6 (4:3), 144:36 (four:1) and 108:36 (three:1), and three corresponding proportions; arithmetic, geometric and harmonic. The kickoff of these proportions is the arithmetic proportion. Here, the second term exceeds the kickoff by the same corporeality every bit the third exceeds the 2d, such equally 2:iii:four or 2:four:vi. The second proportion is called geometric. Here the first term is to the second equally the second is to the third, for example 1:2:four or 1:3:nine. The final proportion is called harmonic. This can exist described when the distance of the two extremes from the hateful is the same fraction of its own quantity. For example 3:4:six, the mean 4 exceeds 3 by one-third of 3 and is exceeded past ane-third of 6. These musical ratios and proportions directly informed the aspiration of architectural class.
In the wake of Pythagoras, Athenian philosopher Plato, in approximately 360 BCE wrote Timaeus, in which he attempted to explain how the universe and everything in it came into existence. Past applying proportion to the solids, he imagined all affair existence built up of five regular bodies; earth was associated with the cube, air with the octahedron, water with icosahedron, fire with tetrahedron, and the trunk of the universe with a dodecahedron. These geometries linked the imperfect physical world with the perfect world of ideas. They illustrated how solid volumes and net shapes, equilateral triangle, foursquare and pentagon, impose rationality onto a whole.
Pythagorean-Platonic tradition laid the foundation for Euclid'due south xiii-book mathematical treatise titled Elements, published around 300 BCE, which gathered and systematically proved all that was known most geometry. In Book VI, definition 3, Euclid cutting a straight line AB in farthermost and mean ratio at C, later to be known every bit the Golden Section, in which the smaller part is related to the larger as the larger is to the whole, in other words AB:Air-conditioning = Air conditioning:CB.
Greek mathematics informed all European systems of proportion that followed. The introduction of the human figure as a proportional reference by Roman engineer Vitruvius, in the outset century BCE, added rational dimension to the notion of one and the whole. Introduced in his Latin treatise, De architectura, the simple picture of square and circle overlapping human proportions strengthened the conviction that basic order and harmony lie deep in human nature.
The principles of proportion were adopted throughout the Byzantine Empire and Middle Ages, through to the Renaissance and Baroque. It is in this context that we notice Märkli'southward hand-drawn studies. Proportion lost significance during the eighteenth century, when it became a matter of individual sensibility and in this respect the architect caused complete freedom; an attitude to which nearly architects operate today [two].
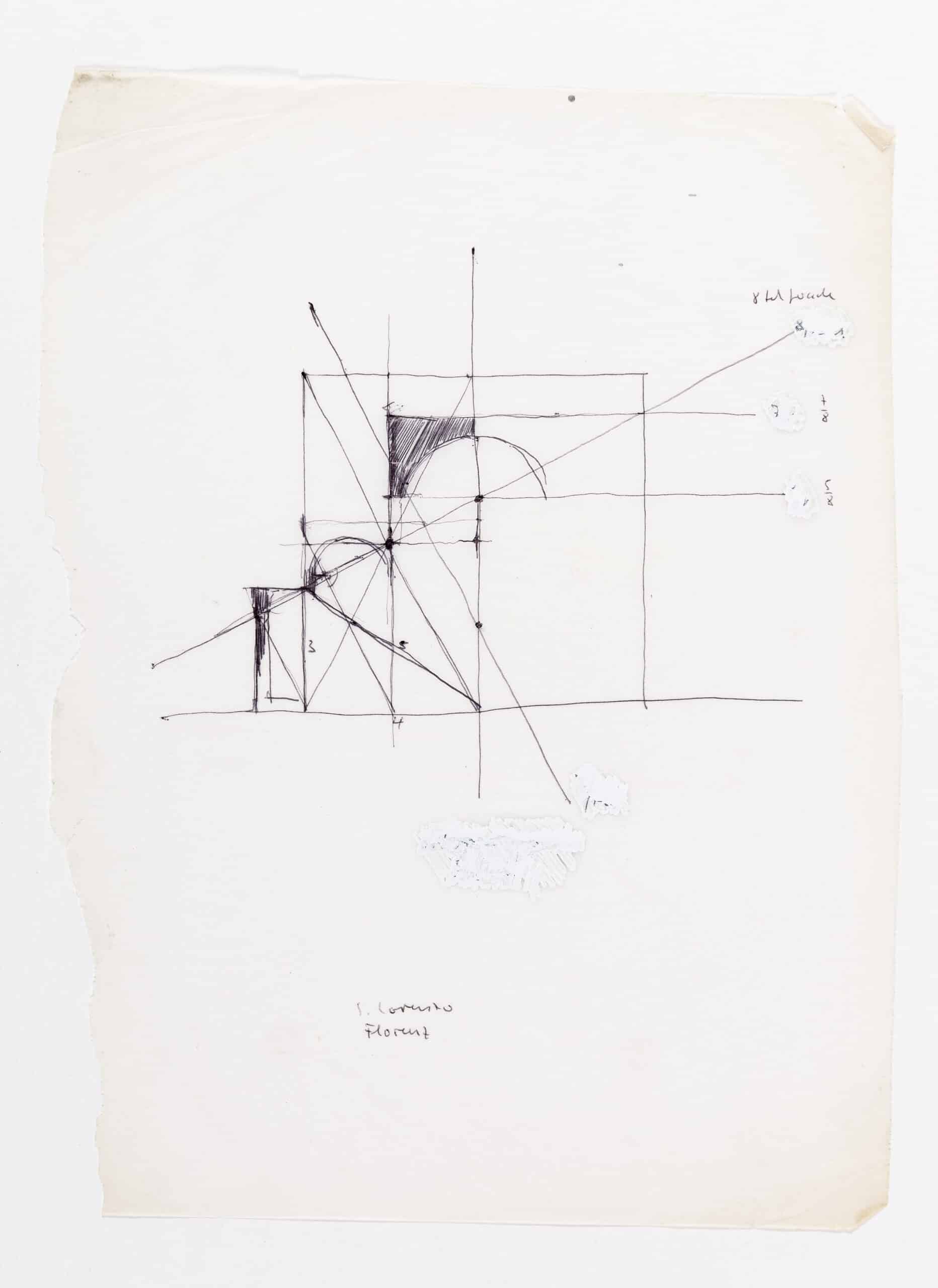
Märkli counters the lost engagement with proportions in twenty-first-century architecture, with an musical instrument that unites a system of proportion learned through observations of history, with a arrangement of measurement based on geometry and human scale. This is presented in his ballpoint-pen tracing of the Byzantium monument Hagia Sophia.
The layered paradigm draws lines, shapes and points on height of the historic precedent to communicate certain ratios, and a method of proportion. Interpreting the lessons set out by Vitruvius, Märkli overlaps foursquare and circle to frame the key volume of the building. The circumvolve intersects the bottom of the square and two upper corners. Its centre is derived from the intersection of two secondary lines that are fatigued perpendicular to the midpoints of two main lines. First, the line for the base of the square, and second, the diagonal line connecting the midpoint of the square's base to the summit left corner. The second perpendicular line intersects the square in a ratio of seven/8 to i/viii on the correct side, and v/8 to 3/8 on the left side. 5/viii corresponds with the Gilt Section down to 7/1000ths, and 7/eight relates to the sine ratio of an equilateral triangle within 9/1000ths. This highlights two cardinal components of proportion attested to throughout history [3].
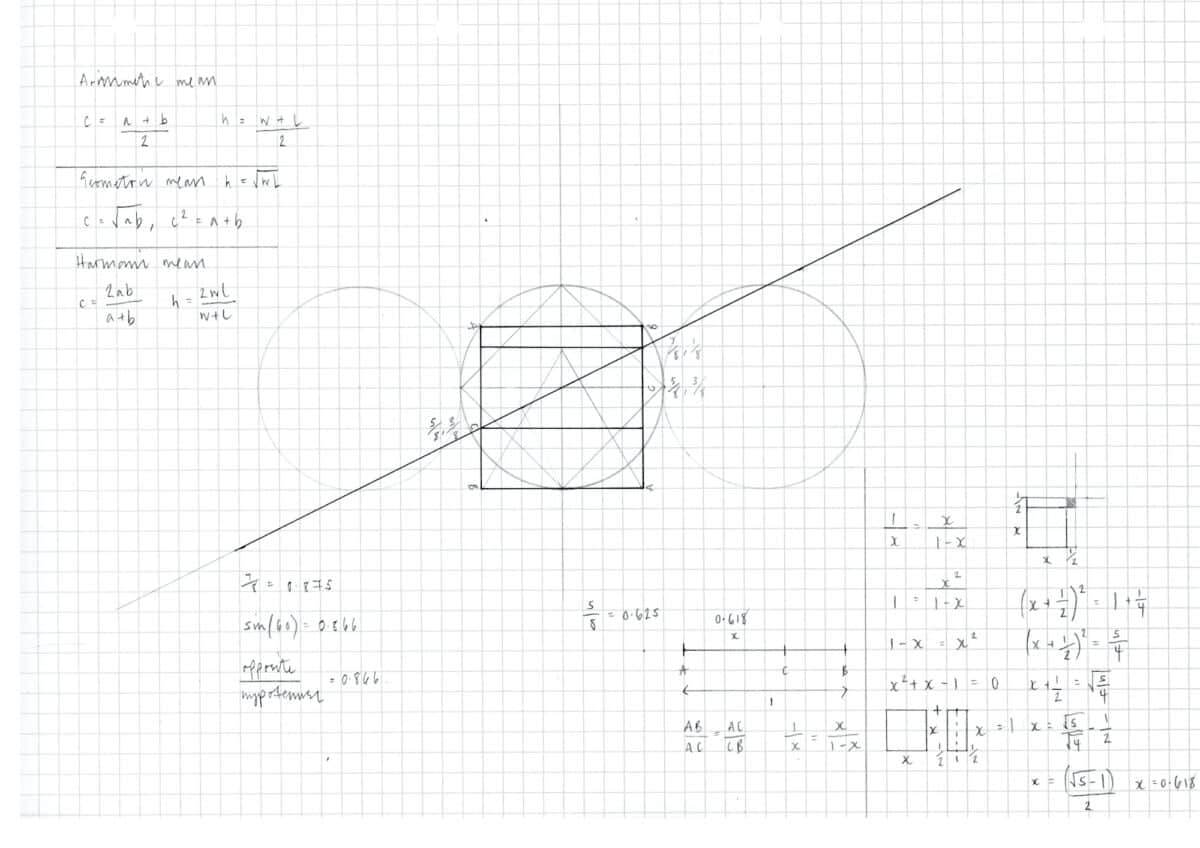
The exclusive nature of the cartoon, overlaid by the symmetrically scalable 8 unit square, reveals the unit length and height of the central book. Length is 8, and height is 5 to the base of dome, and vii to the height of dome. However, for true proportion there must exist a third dimension, in this case the width is missing. This can be calculated using the iii types of proportion established by Pythagoras in music and adult during the Renaissance. When the height of the room is understood to be the hateful, 5 or 7, the width is 2 or 6 in arithmetics proportion; iii.125 or 6.125 in geometric proportion; 3.63 or half dozen.2 in harmonic proportion [4].
Surveyed plans of Hagia Sophia reveal the primary infinite width is roughly 3.65, 2/100ths shy of three.63. This reveals the volume, to the base of operations of dome, is harmonically proportioned and implies Märkli uses this type of proportion as an ideal when designing [v].
The language of architecture illustrated is not a return to antiquity, it is the art of historical translation, and so to imagine something new for the hereafter using a universally valid method. Proportion and grammer are tools to communicate – a means of taming the capricious and chaotic with an social club and structure that can be traced back to our oldest ancestors.
Stacey Lewis is currently undertaking her Role 3 architectural studies in London.
This text was entered into the 2020 Cartoon Matter Writing Prize.Click here to read the winning texts and more writing that was particularly enjoyed by the prize judges.
Notes
- The introduction is derived from ideas in the following source: Imoberdorf, Chantal. Märkli: Chair of Architecture at the ETH Zurich 2002-2015. Zürich: Gta Verlag, 2016.
- The historical context department refers to dates and ideas written in the following sources: Fletcher, Banister. A History of Compages. London: Routledge, 1996, twentieth edition; first published 1896; Wittkower, Rudolf. Architectural Principles in the Historic period of Humanism. Chichester: Academy Editions, a division of John Wiley & Sons, 1998, fifth edition; first published in 1949.
- This paragraph paraphrases Märkli, Peter. Interviewed in: Gerber, Andri., Joanelly, Tibor., & Atalay Franck, Oya. Proportions and Cognition in Architecture and Urban Design: Measure out, Relation, Analogy. Berlin: Dietrich Reimer Verlag, 2019, pp.118-119.
- Hateful formulas: arithmetic mean h = w+fifty ÷ two, geometric mean h = wl, harmonic mean h = 2wl ÷ west+l, where h is the peak, l the length, and w the width of room. Formulas cited in: Mitrovic, Branko. Palladio's Theory of Proportions and the Second Book of the Quattro Libri dell'Architettura. Periodical of the Club of Architectural Historians, September 1990, p.279.
- Hagia Sophia dimensions taken from: Fletcher, Banister. A History of Compages, p.303.
Source: https://drawingmatter.org/the-language-of-architecture-peter-marklis-system-of-proportion/
0 Response to "A Rational System for Creating Order and Perfect Proportion in Architecture and Art Quizlet"
Post a Comment